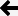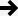The KRAGTEN spreadsheet approach for uncertainty calculation

This is a numerical method, suggested by Kragten which makes effective use of spreadsheet software to provide a combined standard uncertainty from input standard uncertainties and a known measurement model.

Spreadsheet software can be used to simplify the calculations of partial differentials. In this sense, the procedure takes advantage of an approximate numerical method of differentiation, and requires knowledge only of the calculation used to derive the final result (including any necessary correction factors or influences) and of the numerical values of the parameters and their uncertainties. The description here follows that of Kragten.

In the expression for u(y(x₁, x₂...x_n))

provided that either y(x₁, x₂...x_n) is linear in x_i or u(x_i) is small compared to x_i, the partial differentials (∂y/∂x_i) can be approximated by:

Multiplying by u(x_i) to obtain the uncertainty u(y,x_i) in y due to the uncertainty in x_i gives

Thus u(y,x_i) is just the difference between the values of y calculated for [x_i+u(x_i)] and x_i respectively.

The assumption of linearity or small values of u(x_i)/x_i will not be closely met in all cases. Nonetheless, the method does provide acceptable accuracy for practical purposes when considered against the necessary approximations made in estimating the values of u(x_i).

See an example of the general scheme of the Kragten spreadsheet in Microsoft Office Excel format.